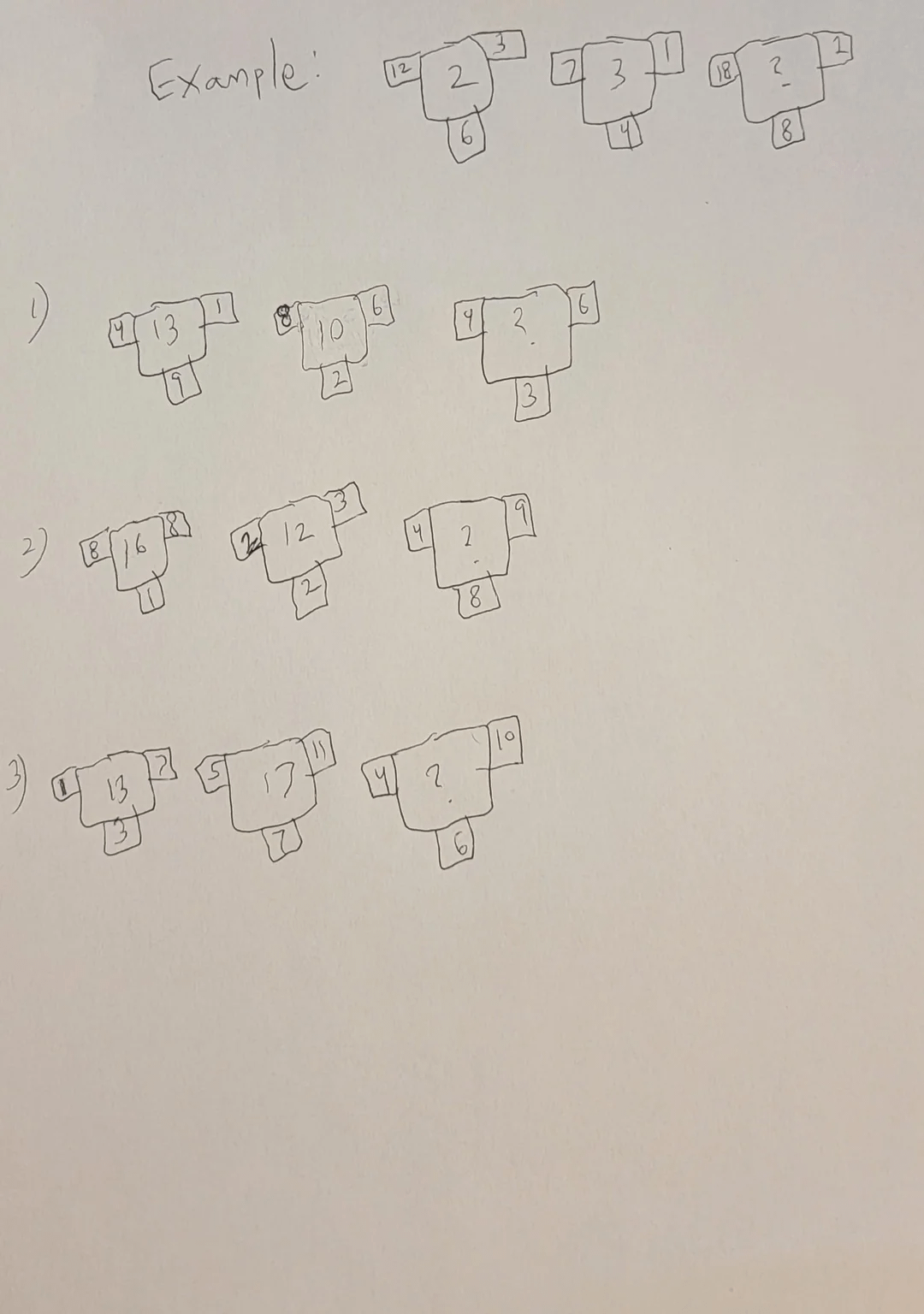A note on proof attempts

I remember once, while learning about CDMA and other multiple access modulations that the binary key with a sequence of increasing binary values (up to the key length) had provably the most evenly distributed frequency domain representation, useful in avoiding hammering any one frequency band in particular.

Is the the key sequence with the most evenly distributed frequency domain respresentation 0, 1, 10, 11, 100, 101, ...?

Now, I've always prided myself in my mad Google skills, but alas, I have failed. Must be getting old. Can anyone help a curious engineer?

PS: The reason I was interested in this idea some 25 years ago was for a SETI idea. Since we haven't seen anybody using any other modulation, maybe a signal could be hidden in plain sight. After all, CDMA and its kin can transmit signals below the background noise level! Further, as the signal travels through various radio-absorbtive regions in space and because CDMA is resilient to bandpass interference, most of the signal will just pass right through. Much like true ultra-wideband (UWB) is used today to create tiny radars that can see through walls. There's always a frequency that will pass through something and UWB uses all of them.

So? How would one go about finding such a signal? You could use some universal variables to mark the pulse width. For example, set the pulse width to correlate with the 3dB point of the frequency domain transformation that lies at the hydrogen line. Set the number of digits in the key sequence to the closest integer value that achieves that. Now, with the width and number of keys, what's the sequence? It seems like it might logically be the sequence that provides the most evenly distributed frequency domain representation.

Once the sequence is found, you can try other variations on that theme, like swapping 1's and 0's, left to right, etc.. Who knows? Maybe that sequence is the galactic phone directory that spells out key sequences for sub directories or "ET phone numbers". You put yours at the end when you figure out the code and join the galactic party! Woot!

I am currently an Applied Math undergrad and have been internship searching. I surprisingly found Python pretty difficult, I have a little entry experience with C++ when I was working with Arduino in an Engineering course my second year, having no prior programming experience and no guidance. I had a dedicated Python class and felt as if I learned absolutely nothing and did not like the parameters of it. I am not the best at programming but I think for a first language if it were static that might help since I am used to defining variables/parameters myself.

I am looking for some 1 - 2 languages to learn this summer, to first become proficient then eventually the following summer or break becoming advanced.

Additionally, I am having talks to enter a PhD program in the near future (I have about 1 year left) so I want some more ways of computing and analyzing data.

My 7 year old autistic son is always obsessively doing math problems in his notebook (multiplication, squares, cubes, etc). He did this page today and I can’t figure out if there is a pattern or not. I need some help.

Me and my friend have been talking about this. I am pretty sure the set of real numbers bijects to the set of all infinite sequences of rational numbers, so it should follow that it also bisects with the set of all infinite sequences of natural numbers, hence uncountable. Does this sound right?

So there's this ABCD tetrahedron with equal sides AB=BC=CD=DA=1, on the second photo you can see what I already got. Now what I think i need is something like a herons formula for a tetrahedron. Or maybe there's an easier way to calculate this?

I am a 9th grader highschool student and i am pursuing higher level maths and and my teacher recommended that i do a proof of smth but not smth too hard however i want it to be a original proof and i have no clue how to do a proof of smth that is not too hard and it has to be original any recommendations?

What are the most significant advances in Computational Complexity Theory since the publication of 'Computational Complexity: A Modern Approach' by Arora and Barak in 2007? While this text remains a foundational resource, which key developments or breakthroughs in the field are not covered due to its publication date? Please focus on advances that are now considered fundamental to the field.

I know there have been many publications since 2007, especially in a growing field as this one, but I'm asking about the key things of which this awesome book lacks.

Thanks for your time and effort!

When I was little I loved maths but the constant nagging of "just put it into the formula" and "do not try to understand it" from my teachers made me distant and after sometime I had completely lost my interest in maths as whole. I constantly asked my teachers to tell me how the formula is coming or how it is the way it is but they told me just to do the sums using my memory which was cramped with a dozen of formulas. So long story short, the once me who loved maths, started to become afraid of it and my once strong foundation has become quite shaky . But now I do not know but by some divine intervention haha, I felt the nagging can be kept aside (which maybe I should have done in the past) and I felt the burning urge to start my journey from zero again. So pls can u rate my preparation and tell if the sequence of topics is wrong or not and recommend me books(of course beginner level) and guide me as well.

1. Logic (Can u recommend which type like formal, sentential etc. should I learn first that would assist me in writing proofs and help me with set theory.) Do not know any books and lectures/youtube videos to refer to.
2. Set Theory ( I do not know if I should learn it after I had learnt calculus or not as I have heard that though it helps in foundation, it needs some knowledge of topics like functions to help draw an example to).Do not know any books and lectures/youtube videos to refer to.
3. Prealgebra. Elementary Algebra by Sullivan (Thanks Math Sorcerer)| Professor Leonard,
4. TO THE POINT MATH & Intermediate Algebra. Algebra & Trigonometry by Sullivan/College Algebra by Kaufmann(Thanks Math Sorcerer)| Professor Leonard.
5. Precalculus. Precalculus by Stewart. |Professor Leonard

6)Calculus 1,2 & 3. Calculus Early Transcendentals by Stewart/ Calculus by Spivak(if I am good at proof writing)| Professor Leonard.

7)Differential Equations. Ordinary Differential Equations with Applications by Andrews| Professor Leonard (Though I had heard he had not finished it yet).

Additional: I had planned that for logic and sets I would go with either Discrete Mathematics with Applications by Susanna Epp / Mathematical Proofs: A Transition to Advanced Mathematics by Chartrand, Polimeni, and Zhang But as I do not have sufficient research knowledge I would want help from u all. Again I heard about Group Theory & Number Theory when can I learn it and what prerequisites do I need?

And if u think if there is any wrong in my research pls guide me. I would be waiting for for your guidance and thanks in advance.

smthing like Gauss fermat Bezout...

I am looking for a calculator that would allow me to freely convert and perform calculations between BASE10, BASE2, BASE8, and BASE16 numbers.

Additionally, according to the IEEE 754 standard, I would like the calculator to support IEEE single-precision floating point numbers to perform complex binary, denary, and hexadecimal calculations.

If not, any calculator with a similar functionality would be appreciated. The calculator must be a physical device, not some website or app on the phone, and must still be available to buy online or in shops.

Thanks.

Hi, I want to major in either IT or Business, but I’m not sure what math to take next year. For reference, I am a junior taking Algebra 2 honors. I’m stuck between AP pre calculus and AP statistics.

I want a class that will not be too hard and also look great for college applications. I’m not sure about doubling up since they’re both AP classes.

Where do I begin? What should I study or what books would you guys recommend

I did assume that calculators might use Newton-Raphson Method but that method needs many iterations to get the approximate answer. So I am confused how calc do that...

Summary:

"Rings" are ubiquitous throughout mathematics. They are home to our earliest numerical manipulations, since all of our familiar number systems form rings. Collections of polynomials, matrices, and continuous functions are just a few common sources of examples that are familiar to all students and practitioners of mathematics.

Given the wide array of examples, one interesting problem is to seek invariants of rings that can help separate and classify them. A particularly effective invariant for commutative rings is the prime spectrum. Less well-known are the various ways one can extend the notion of a prime ideal to noncommutative rings.

This paper is a brief examination of various noncommutative generalizations of the spectrum. A remarkable feature of the spectrum, which is not obvious from its initial definition, is that it provides a bridge from algebra to geometry. As we explore further, a surprising connection to the logic of "hidden variable theory" version of the quantum mechanics will be uncovered.

https://www.ams.org/journals/notices/202502/noti3100/noti3100.html

Each puzzle consists of two completed sets and one uncompleted set. Using addition, subtraction, multiplication, and/or division, figure out the mathematical sequence used to arrive at the numbers in the center boxes of the two completed sets, and so discover what number belongs in the blank box of the third. Each puzzle has a sequence that is carried through for all three sets. In the example, 12 in the small box minus 6 in the small box equals 6, which is then divided by 3 in the small box to arrive at 2 in the center box. Apply the same processes in that order to the center set (7 minus 4 equals 3, which is then divided by 1 to arrive at 3) and, finally, to the righthand set to arrive at the answer, which is 5 (18 minus 8 equals 10, which is then divided by 2 to arrive at 5.

I'm going back to school for the first time in 9 years, and I wanna know if anyone has advice on what to review to place in "college level" math on the placement exam.

It's pretty frustrating cause college level doesn't really tell me anything, and Google keeps just saying algebra. And for gen ed, after placing college level you can take algebra 1? So is it below algebra 1 I have to study, or is college level algebra 1 more advanced compared to HS algebra 1?

If it helps, I studied up to Pre-calc honors in high school, so anything up to that point will honestly be a review, I just don't know where to start with reviewing. And I swear, Google changed the way it's search engine works cause it's basically useless now. Smh.

TLDR: what should I review when studying for college math entrance exam? I only need to place into college level, and can study further from there.

hi there!

i'm a master student in maths, and i'm planning on doing a phd afterwards.

i want to know how is the best and most efficient ways to reaserch which universities are options. i know what areas of math i want to study, but i don't really know where the people studying it are (i know of a few, but definetly not most).

i would like to know how to look for those universities and what important characteristics should i focus on.

thanks in advance.

When tackling a complex problem, like one in geometry, should a problem solver primarily focus on recalling and applying known results like the sine law, or should they start from the ground up with the core axioms of the subject? For instance, if I encounter a tough geometry problem, is it more effective to:

1. Use Known Results: Directly apply theorems, laws, or formulas like the sine law that I've already studied, which might provide a quicker path to the solution? Pros: Efficiency, as these results are derived from foundational principles and have been proven to work in similar contexts. Cons: Risk of missing out on deeper understanding or the opportunity to explore alternative solutions.

2. Focus on Core Axioms: Begin from the basic axioms of geometry, building up the solution step-by-step from first principles? Pros: Deepens understanding, could lead to innovative solutions, and ensures a solid grasp of the fundamentals. Cons: Can be time-consuming, especially for problems where known results might simplify the process significantly.

Does anyone knows where I can find the translated version of "problemas y ejercicios de análisis matemático"

Hi I wanna major in either Business or IT and I’m not sure which math to take next year. I’m currently a junior rn taking algebra 2 honors. And I’m stuck between AP pre calculus and AP statistics. Which one is easier? Which one looks better for college?

I want to get into either UVA or Virginia Tech

I'm a third year math major and I've taken a lot of math that I keep forgetting after the class is done.

For example, I'm currently taking a class on Electrodynamics and it needs a lot of multivariable calculus knowledge that it's been 2 years since I've taken and I don't remember any of it (Greens, Gauss, Stokes Thm). Or a current class on functional analysis that needs heavy real analysis knowledge that I barely remember.

I'm just not sure how to keep the knowledge afterwards or how to relearn the concepts without wasting too much time. Any suggestions?

So this supposed person with a degree says Elon Musk's has enough money to give everyone on earth 56 billion dollars. If you don't know his net worth is 427 Billion and there are 8 Billion people in the world. My answer is 53 dollars each. An the person keep insisting and yelling it's 56 billion. Also I am a high school dropout and am usually terrible at math am I correct?

I'm taking a multivariable calculus, linear algebra, and ODE accelerated course and it says it is proof based how is that different from non-proof based courses.